| MULTIPLICATION |
What is Multiplication?
Multiplication is actually repeated addition.
So, 4 times 3 tells us to add four 3's and 2 times 5 tells us to add two 5's.
4 × 3 = 3 + 3 + 3 + 3
2 × 5 = 5 + 5
However, finding sums rather than products is not very efficient when we want 36 times 9. First of all we'd have to turn our paper sideways to write thirty-six 9's and all those plus signs, then it would take us a long time to find the sum. So, instead of making life difficult for ourselves, we learn the multiplication tables and we learn them well.
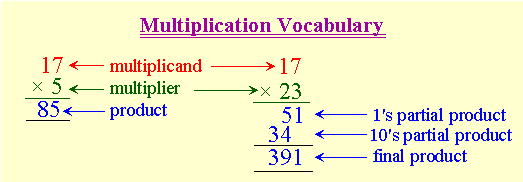
Multiplier, Multiplicand and Product
The result (answer) of multiplication is called the product. The number doing the multiplying is called the multiplier (what else?). The number being multiplied is called the multiplicand, but if we don't know our multiplication tables, it's called the MULTIPLI-CAN'T! If a word problem says " the product of 2 numbers is 24 ", we know the numbers were multiplied to give 24, because we see the word product.

Multiplying and Carrying
Since multiplication is addition, and when we add, we often have to carry values from one column to the next -- we do the same when we multiply. If the product of any column is bigger than 10 times the value of the column, we carry to the neighboring place on the left.
Say we're multiplying 6 × 14.
We start with the one's column: 6 × 4 = 24
We put 4 in the 1's column of the product, then carry 2 tens to the ten's place.
Then, we multiply 6 × 1 ten = 6 tens, add the 2 tens we carried to get 8 tens.
The product of 6 × 14 is 84.
Watch for this OOPS! A common mistake for beginners is to multiply the carried number instead of adding it. We must remember that we ADDED THE CARRIED VALUE TO THE COLUMN -- so first we multiply and then we add what we carried.

When the multiplier is only 1 digit, we can use an approach like the Column Sum approach to addition called the Column Product Method in which we write the product of the multiplier and each column with the columns aligned and then we add.

.
Two-Digit Multipliers
Let's do an example to explain the difference between 1-digit and 2-digit multipliers.
It's just a matter of lining up the partial products according to place value.
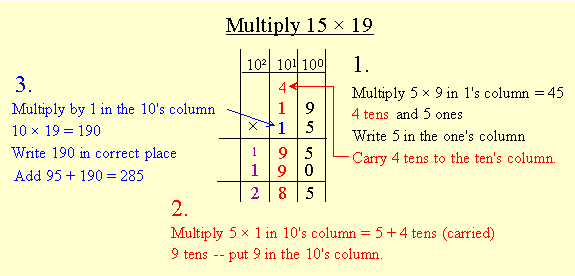
Notice the " 0 " in the one's column in the 2nd line of the partial product. In the third step, we're multiplying by 1 -- but it is in the TEN'S COLUMN so we get 19-tens = 190. We sometimes omit the zero (like in this example on the right) because it is understood, but we always move the 2nd line of the partial product over one column to the left. With a 3 digit multiplier, the 3rd line of the partial product would either have 2-zeros at its right end, or, the last digit in this line would be under the hundred's column.
.
Estimating the Product: making an educated guess
Often, if we're not sure our product is correct, or when we want to know approximately what the product will be, we can make an "educated guess" or estimate. We round the numbers up or down, then multiply them, to determine about how much the answer should be.
In the last example, we had 19 × 15.
300 is a good estimate here because it is
20 × 15, and 20 is very close to 19
Since 15 is exactly half way between 10 and 20, rounding it up to 20 or down to 10 makes our estimate much less exact. And since it's easy to multiply by 15, we didn't round it.
That was by choice, but if we follow the "rounding rules" precisely, our estimate
becomes 20 × 20 or 400. Our first estimate -- 300 -- is only 15 more than the actual answer but
400, the estimate we get when we follow the rules, is 115 more than 285, so in this case it is better not to round. If the 15 was 14 or 16, we would round 14 down to 10 and 16 up to 20.
![]()
Now get a pencil, an eraser and a note book, copy the questions,
do the practice exercise(s), then check your work with the solutions.
If you get stuck, review the examples in the lesson, then try again.
Practice
1) Round these numbers, estimate the product, then multiply to find the product:
a)  |
b)  |
c)  |
d)  |
2) Use the Column Product Method to multiply:
a)  |
b)  |
c)  |
d)  |
3) Find each product. Carry and put zeros in columns where needed.
a)  |
b)  |
c)  |
.
![]()
Solutions
1) Round these numbers, estimate the product, then multiply to find the product:
a)  |
estimate 30 × 10 = 300 multiply: 34 × 8 = 272 |
b)  |
estimate 600 × 10 = 6000 multiply: 576 × 7 = 4032 |
c)  |
estimate 50 × 100 = 5000 multiply: 97 × 52 = 5044 |
d)  |
estimate 700 × 50 = 35 000 multiply: 737 × 46 = 33 902 |
2) Use the Column Product Method to multiply:
a)   |
b)   |
c)   |
d)   |
3) Find each product. Carry and put zeros in columns where needed.
a)   |
b)    |
c)    |
![]()