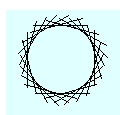
WHAT'S A TANGENT?
In the fall of 1995, I was speaking to a producer at CBC Radio who was preparing me to participate in a coast to coast discussion on wild birds. I was to speak after two other bird watchers. The producer was trying to explain the dynamics of the discussion. She said: " While the other two are speaking, you'll be able to hear them -- but they won't be able to hear you, so you can't say anything to them -- so don't try to participate in the discussion while the others are speaking." Then she paused, sighed and said: "It's pretty complicated, I know ----"
I cut her off and said: "It's not complicated for me. I teach college math. I understand perfectly what you said."
She took a breath and blurted out "What's a tangent???" Then she caught herself and apologized for being so direct. She explained that all her life she'd wanted to know what a tangent is -- and no one could ever explain it so she could understand it.
I assured her that I always welcome the opportunity to teach someone -- anyone actually -- about math. See, I figure, the more people who understand the math all around us, the better the drivers will be. A knowledge of geometry makes one a better driver, and bowler, and budgeter etc. -- but back to the tangent.
I said: "Have you ever cut out a circle with a scissors?"
She said: "Yes."
I asked: "How can you do that? A scissors can only cut a straight line."
Then I asked: "Have you ever driven a traffic circle?"
She said: "Yes."
I asked: How can you do that? A car can only drive a straight line."
Then I asked: "Have you ever seen someone riding the waves on a surfboard?"
She said: "Yes."
"How can that be?" I asked. "A surfboard is a straight line. A wave is a curve."
By then, she saw the picture. A segment of a tangent is what you cut, drive, or surf. The tangent in math actually touches the curve in a single teeny-tiny point, but humans can't cut, drive or surf that precisely, so we actually use a segment of the tangent line. We've all seen that string artwork which defines a circle using straight lines. Those lines are tangents. So tangents are essential to the shape of the curve.
This is why Isaac Newton and his buddies invented Calculus. That's why we study derivatives. Let's think about that word for a minute. It stems from "derive" which means: to obtain or draw from a source. In other words, when we find a function's first derivative, we get information about the tangents that define the curve of the function -- the lines from which the function's curve is derived.

tangents
.
TtT
.