solving word problems with math

SOLVING WORD PROBLEMS WITH MATH

Where To Start -- What to Do

Solving a math question is like knowing where you are, knowing where you want to be, and finding the way to get there from here. Though that's true about life in general, in Math, there's one extra constraint on the action. In Mathland, you must find THE BEST WAY -- THE MOST EFFICIENT AND PRECISE WAY -- to get to there from here. So doing a math question can be compared to having to find a specific path of stepping stones across a wide river -- a path which begins on the stone where you are on this side of the river, ends where you want to be -- on the last stone -- on the other side of the river -- when all the stones in between are unknown -- they have ?s on them. The objective of the exercise is to find those unknown stepping stones.

In math, as in life, there are often many ways to get to where you want to be from where you are, but in Mathland, your mission is to find the straightest road between the two. You're looking for a bee line from here to there. You're not looking for the scenic route -- you want the autoroute -- the expressway. So how do you find it? You can't look at a map like you would if you were going from one city to another. What you must do is find the right combination of given facts and previously learned properties, theorems and axioms which will lead to the solution of the problem.

From Diagram to Equation to Solution

Let's solve this problem together, so get paper and pencil right now and we'll work it out.

A farmer buys 1000 yards of fencing to enclose a rectangular field. If he wants the length
to be 3 times the width, what dimensions should he make the field?

First, we underline and list the data or information we learn from the words.
Also, whenever we can in math, we make a diagram! It really helps!!

The data or information list is:

1) a rectangular field

2) length is 3 times width

3) fence = perimeter = 1000 yards.

Now for the diagram:

Draw a rectangle to represent the field. We know that in a rectangle, opposite sides are equal, and we know that in this rectangle, the length = 3 times the width. Our diagram should indicate this, so we use w to represent the width of the field, and 3w to represent the length; then we put w and 3w on opposite sides of the rectangle in our diagram. Now, we know the perimeter of a rectangle is twice the sum of the width and length, so we can express the Perimeter of our field as

P = 2 ( w + 3w) or P = 2 (4w) = 8w.

The question said that the perimeter is 1000 yards so we write: 8w = 1000
and when we divide both sides of the equation by 8, we get
w = 125 and therefore 3w = 375
The field is 125 yards wide and 375 yards long.

Since we are solving a word problem, it is proper protocol and convention to state the solution in words. This question asks us to find the dimensions of the field. It did not ask us to find w. We chose w as the variable to represent the width, then we used it to solve an equation, but we have to finish the question with a sentence that answers it. That's why we include the units (yards) in our answer statement and that's why we answer with a sentence.

We can verify our solution by checking if indeed the fence around the field measures 1000 yards and one side is 3 times the other. Since 2 (125 + 375) = 1000, and 375 = 3 × 125, we have solved this word problem correctly.

To find the solution, we used the 3 facts given in the question: ie. that the field is rectangular, it has a perimeter of 1000 yards, and the length is 3 times the width. We made a picture of the situation, labeled it with constants and variables to represent the given information, then we used the formula for perimeter to make an equation or algebraic statement about the dimensions. Once we solved the equation, we answered the question. Therefore we found the most direct route from where we were to where we wanted to be. This is what all math questions are about -- finding the logical straight line of thought that leads from what we know to what we want to know or prove. Efficiency and Precision -- that's the MathWay.

Did your diagram and solution look like this?

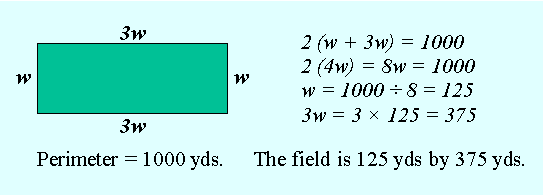
Now get a pencil, an eraser and a note book, copy the questions,
do the practice exercise(s), then check your work with the solutions.
If you get stuck, review the examples in the lesson, then try again.

Practice Exercises

For each of these word problems, make a data list,, write an equation and solve.
If the situation allows, make a diagram. Remember to answer with a sentence.

1) Maria's Granny gave her some money for her birthday so she went shopping for some new clothes. She bought 3 blouses on sale at $7 each and a pair of jeans for $19. If the price includes the tax and Maria had $15 left over after she paid, how much did her Granny give her for her birthday?

2) Jonathan and James were talking about their baseball card collections. James said: "yesterday, I started out with the same number of cards as you have now, but I traded 6 of my cards for 9 of George's. If together they have 35 cards now, how many cards does James have now?

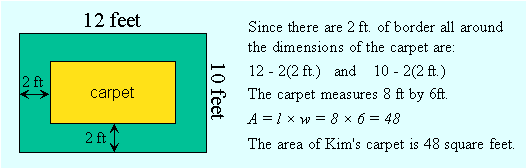
3) Kim's parents decide she needs a carpet for her bedroom floor to keep her tootsies warm when she's in bare feet. Her room measures 10 feet by 12 feet, but they want a carpet that leaves a border of floor showing between the wall and the carpet on all 4 sides.
If the width of the border is 2 feet, what is the area of the carpet they get for Kim's room?

4) Harriet's house has the biggest back yard anyone has ever seen. It measures 121 yards by 80 yards. Her friend Jennifer thinks the area is more than 3 acres. If there are
4 840 square yards in an acre, is Jennifer correct? How many acres does Harriet's back yard cover?

5) If the approximate length of the earth's orbit around the sun is 583 400 000 miles a year,
a) how many miles does the earth travel in a month?
b) how many miles does the earth travel in a week?
c) how many miles have you travelled since you were born?
(use your age in years, months and weeks)

Solutions

1) The data or information list is:

1) 3 blouses at $7 Solution:
Let M represent the how much money Maria got from Granny
blouses cost $21, jeans cost $19, she had $15 left
so, 3(7) + 19 + 15 = M which means 21 + 19 + 15 = 55
Granny gave Maria $55 for her birthday.

2) jeans for $19

3) $15 left over

2) The data or information list is:

1) yesterday they were equal Solution:
Let J represent the number of cards Jonathan has today
James had J cards yesterday, traded 6 for 9
so, J + J - 6 + 9 = 35 which means 2J + 3 = 35
now subtract 3 and divide by 2 on both sides to get J = 16
James has 19 cards today -- 3 more than Jonathan.

2) James gave Jack 6 cards

3) James got 9 from Jack.
4) total of 35 today

3) The data or information list is:

1) floor 10 ft × 12 ft

2) border 2 ft

4) The data or information list is:

1) 121 yards by 80 yards Solution:
A = l × w so the yard's area = 121 × 80 = 9 680
we divide by 4 840 to find acres so 9 680 ÷ 4 840 = 2
Jennifer was wrong. The area of Harriet's yard is 2 acres.

2)4 840 yds² in an acre

a) 12 months in a year: 583 400 000 ÷ 12 = 48 616 666.67 miles.
The earth travels 48 616 666.67 miles in a month.

b) 52 weeks in a year: 583 400 000 ÷ 52 = 11 219 230.77 miles.
The earth travels 11 219 230.77 miles in a week.

c) (your age years × 583 400 000) + (your age in months × 48 616 666.67) +
(your age in weeks × 11 219 230.77).

( Algebra Mathroom Index )

1) 3 blouses at $7	Solution: Let M represent the how much money Maria got from Granny blouses cost $21, jeans cost $19, she had $15 left so, 3(7) + 19 + 15 = M which means 21 + 19 + 15 = 55 Granny gave Maria $55 for her birthday.
2) jeans for $19
3) $15 left over

1) yesterday they were equal	Solution: Let J represent the number of cards Jonathan has today James had J cards yesterday, traded 6 for 9 so, J + J - 6 + 9 = 35 which means 2J + 3 = 35 now subtract 3 and divide by 2 on both sides to get J = 16 James has 19 cards today -- 3 more than Jonathan.
2) James gave Jack 6 cards
3) James got 9 from Jack. 4) total of 35 today

1) 121 yards by 80 yards	Solution: *A = l × w* so the yard's area = 121 × 80 = 9 680 we divide by 4 840 to find acres so 9 680 ÷ 4 840 = 2 Jennifer was wrong. The area of Harriet's yard is 2 acres.
2)4 840 yds² in an acre